2018 A-level H2 Mathematics (9758) Paper 2 Suggested Solutions

All solutions here are SUGGESTED. Mr. Teng will hold no liability for any errors. Comments are entirely personal opinions.

Numerical Answers (click the questions for workings/explanation)

Question 1: $f(x) = 3 (\frac{2}{9}x + 4)^{3/2} + 45, (54, 237)$
Question 2: $s = 69, t = 13$ , other roots $= 2 + 3i, 0.5$ , $w = - \frac{3}{2} \pm \frac{3\sqrt{3}}{2}i, w_1 w_2 w_3= 27, w_1 + w_2 + w_3= 0$
Question 3: $D(-5, -4, 3), 4x + 45y + 20z = 200, 58.6^{\circ}, 6.88 \text{units}$
Question 4: $\text{ln}(\cos 2x) = -2x^2 - \frac{4}{3}x^4 - \frac{64}{45}x^6, x \neq \frac{\pi}{4}, -1.0644, -1.0670$
Question 5: $k \ge 9430000$
Question 6: $\frac{5}{9} \textless p \textless \frac{2}{3}, 0.43046721$
Question 7: $\text{P}(A' \cap B') = 1 - a - b + ab, \text{P}(A' \cap C') = 1 - a - c, \frac{2}{15} \le \text{P}(A \cap B) \le\frac{1}{3}$
Question 8: $\text{P}(S=10)=0; g(n) = 22n^2 + 78n +36$
Question 9: $r = 0.969, r = 0.993; P = 2.85 \times 10^{-8} R^2 - 0.283, R = 6450 \text{~revolutions~per~min}, P = 0.0273 \text{~watts}, P =1.02 \times 10^{-4} R^2 - 0.283$
Question 10: $0.605, 0.773, 0.126, 137, 0.163$

(i)
$\int (\frac{1}{3}y - 15)^{-1/3}~dy = \int 1 ~dx$

$\frac{9}{2}(\frac{1}{3}y - 15)^{2/3} = x + C$

Sub $x = 0, y = 69, \Rightarrow C = 18$ .

$(\frac{1}{3}y - 15)^{2/3} = \frac{2}{9}(x + 18)$

$y = 3[(\frac{2}{9}x + 4)^{3/2} + 15]$

$\therefore f(x) = 3(\frac{2}{9}x + 4)^{3/2} + 45$

(ii)
$\frac{dy}{dx} = 4 \Rightarrow y = 237$

$(\frac{1}{3}(237 - 15)^{2/3} = \frac{2}{9}(x + 18) \Rightarrow x = 54$

Required coordinates: $(54, 237)$

(a) Since the coefficients of the given polynomial are all real and $x = 2 + 3i$ is a root, we have that $x = 2 - 3i$ is also a root. We have a quadratic factor $(x - 2 - 3i)(x - 2 + 3i) = x^2 - 4x + 13$ .

$(x^2 - 4x + 13)(4x^2 + ax + b) = 4x^4 - 20x^3 + sx^2 - 56x + t$

Comparing the coefficient of $x^3$ , $- 16 + a = -20 \Rightarrow a = -4$

Comparing the coefficient of $x$ , $- 4(b) + 13(-4) = -56 \Rightarrow b = 1$

Comparing the coefficient of constant, $t = 13$

Comparing the coefficient of $x^2$ , $b + 52 + (-4)(-4) = s \Rightarrow s = 69$

$\therefore 4x^4 - 20x^3 + 69x^2 - 56x + 13 = (x^2 - 4x + 13)(4x^2 - 4x + 1)$

$(x^2 - 4x + 13)(4x^2 - 4x + 1) = 0$

$(x^2 - 4x + 13)(2x - 1)^2 = 0$

$x = 2 \pm 3i, x = 0.5$

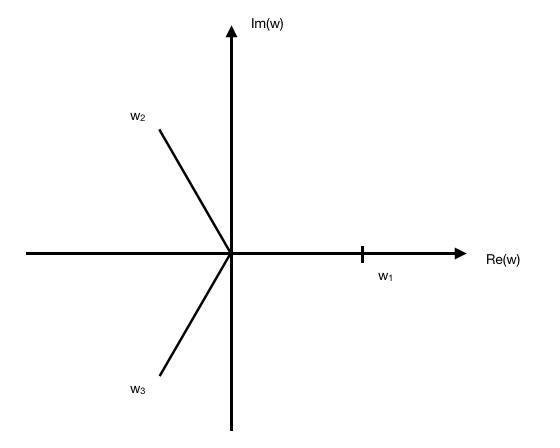
Other roots are $2 + 3i, 0.5$ .

(bi)
$w^3 - 27 = 0$

$(w-3)(w^2 + 3w + 9) = 0$

$w^2 + 3w + 9 = 0 \text{~or~} w = 3$

$w = \frac{-3 \pm \sqrt{-27}}{2}$

$w = - \frac{3}{2} \pm \frac{3\sqrt{3}}{2}i$

Other $w = - \frac{3}{2} + \frac{3\sqrt{3}}{2}i , - \frac{3}{2} - \frac{3\sqrt{3}}{2}i$

(bii)
Using GC,
$w_1 = 3 = 3(\text{cos}0 + i \text{sin}0)$

$w_2 = - \frac{3}{2} + \frac{3\sqrt{3}}{2}i = 3(\text{cos}\frac{2\pi}{3} + i \text{sin}\frac{2\pi}{3}) = 3 e^{i \frac{2\pi}{3}}$

$w_3 = - \frac{3}{2} - \frac{3\sqrt{3}}{2}i = 3(\text{cos}\frac{2\pi}{3} - i \text{sin}\frac{2\pi}{3}) = 3 e^{-i \frac{2\pi}{3}}$

Argand diagram for 3bii

Note: they should be drawn at equal lengths of 3 units and 120 degrees apart from each other.
(biii)
$w_1 w_2 w_3= 27$

$w_1 + w_2 + w_3= 0$

They are the coefficients of $w^3 - 27 = 0$ actually.

(i)
Since ABCD is a parallelogram, $\vec{AD} = \vec{BC}$

$\vec{OD} - \vec{OA} = \vec{OC} - \vec{OB}$

$\vec{OD} = \begin{pmatrix}-5\\-4\\3\end{pmatrix}$

$\therefore D(-5, -4, 3)$

(ii)
$\vec{CE} = \begin{pmatrix}5\\-4\\8\end{pmatrix}$

$\vec{BE} = \begin{pmatrix}-5\\-4\\10\end{pmatrix}$

$\vec{CE} \times \vec{BE} = \begin{pmatrix}-8\\-90\\-40\end{pmatrix}$

$\textbf{r} \cdot \begin{pmatrix}4\\45\\20\end{pmatrix} = 200$

$\pi_{BCE} : 4x + 45y + 20z = 200$

(iii)
$\vec{BC} = \begin{pmatrix}-10\\0\\2\end{pmatrix}$

$\vec{BA} = \begin{pmatrix}0\\-8\\1\end{pmatrix}$

$\vec{BC} \times \vec{BA} = \begin{pmatrix}16\\10\\80\end{pmatrix}$

Required angle $= \text{cos}^{-1}\frac{\bigg| \begin{pmatrix}4\\45\\20\end{pmatrix} \cdot \begin{pmatrix}8\\5\\40\end{pmatrix} \bigg|}{\bigg| \begin{pmatrix}4\\45\\20\end{pmatrix} \bigg| \bigg| \begin{pmatrix}8\\5\\40\end{pmatrix} \bigg|} = 58.6^{\circ}$ (1 DP)

(iv)
Midpoint $\vec{OM} = \frac{\vec{OA} + \vec{OD}}{2} = \begin{pmatrix}0\\-4\\2\end{pmatrix}$

Distance of $M$ to plane is equals to the length of projection of $\vec{BM}$ onto the normal.

$\vec{BM} = \begin{pmatrix}0\\-4\\2\end{pmatrix} - \begin{pmatrix}5\\4\\0\end{pmatrix} = \begin{pmatrix}-5\\-8\\2\end{pmatrix}$
Distance required $= \frac{\bigg| \begin{pmatrix}-5\\-8\\2\end{pmatrix} \cdot \begin{pmatrix}4\\45\\20\end{pmatrix} \bigg|}{\bigg| \begin{pmatrix}4\\45\\20\end{pmatrix} \bigg|} = \frac{340}{\sqrt{2441}} = 6.88 \text{~units}$

(i)
$\text{ln}(\text{cos}2x)$

$\approx \text{ln}(1 - 2x^2 + \frac{2}{3}x^4 - \frac{4}{45}x^6)$

$= - 2x^2 + \frac{2}{3}x^4 - \frac{4}{45}x^6 - \frac{1}{2}(- 2x^2 + \frac{2}{3}x^4 - \frac{4}{45}x^6)^2 + \frac{1}{3}(- 2x^2 + \frac{2}{3}x^4 - \frac{4}{45}x^6)^3 + \ldots$

$\approx - 2x^2 + \frac{2}{3}x^4 - \frac{4}{45}x^6 - \frac{1}{2}(4x^4 - \frac{4}{3}x^6 - \frac{4}{3}x^6) + \frac{1}{3}(-8x^6)$

$= -2x^2 - \frac{4}{3}x^4 - \frac{64}{45}x^6$

$x \neq \frac{\pi}{4}$ for the expansion to be valid since $\text{cos}2(\frac{\pi}{4}) = 0$ , thus $x = \frac{\pi}{4}$ is not in the domain.

(ii)
$\int \frac{\text{ln}(\cos 2x)}{x^2}~dx$

$= \int - 2 - \frac{4}{3}x^2 - \frac{64}{45}x^4 ~dx$

$= -2x - \frac{4}{9}x^3 - \frac{64}{225}x^5 + C$

$\int_0^{0.5} \frac{\text{ln}(\cos 2x)}{x^2}~dx$

$= \bigg[ -2x - \frac{4}{9}x^3 - \frac{64}{225}x^5 \bigg]_0^{0.5}$

$\approx -1.0644$ (4 DP)

(iii)
Using GC, $\int_0^{0.5} \frac{\text{ln}(\cos 2x)}{x^2}~dx = -1.0670$ (4 DP)

(i)
A sample size of 30 will be sufficiently large for the manager to approximate the sample mean distribution to a normal distribution by Central Limit Theorem.

The fans should be randomly chosen, i.e., the probability of a fan being chosen should be equal and the fans are chosen independently of each other.

(ii)
Let $X$ denote the time to failure, in hours

Let $\mu$ denote the population mean time to failure, in hours.

Let $H_0$ denote the null hypothesis.

Let $H_1$ denote the alternative hypothesis.

$H_0 : \mu = 65000$

$H_1 : \mu \textless 65000$

(iii)
Let $k$ be the required variance.

Under $H_0$ , $\bar{X} \sim \text{N} (65000, \frac{k}{43})$ approximately by Central Limit Theorem since the sample size is sufficiently large.

To not reject $H_0$ at 5% level of significance,

$\text{P}( \bar{X} \textless 64230) > 0.05$

$\frac{64230 - 65000}{\sqrt{\frac{k}{43}}} > -1.6448526$

$k > 9423134$

$\Rightarrow k \ge 9430000$ (3 SF)

(i)
Let $X$ denote the number of left fork bug takes.

$X \sim \text{B}( 8, p)$

$\text{P}(X = 5) = {8 \choose 5} p^5 (1-p)^3 = 56 p^5 q^3$

(ii)
For the probability to be greatest, $x = 5$ is the modal value. This means that $\text{P}(X = 5) > \text{P}(X = 4)$ and $\text{P}(X = 5) > \text{P}(X = 6)$ .

$\text{P}(X = 5) > \text{P}(X = 4)$

$56 p^5 q^3 > {8 \choose 4} p^4 q^4$

$56 p^5 q^3 > 70 p^4 q^4$

$\frac{4}{5}p > q$

$\frac{4}{5}p > 1 - p$

$\Rightarrow p > \frac{5}{9}$

$\text{P}(X = 5) > \text{P}(X = 6)$

$56 p^5 q^3 > {8 \choose 6} p^6 q^2$

$56 p^5 q^3 > 28 p^6 q^2$

$q > \frac{1}{2}p$

$1 - p > \frac{1}{2}p$

$\Rightarrow p \textless \frac{2}{3}$

$\therefore \frac{5}{9} \textless p \textless \frac{2}{3}$ .
(iii)
The probability of not entering the black hole $= 0.9$ .

Bug take 8 steps regardless of which fork it wants.

Required probability $= (0.9)^8 = 0.43046721$ (exact)

Required probability $= (0.9p)^8 + {8 \choose 1} (0.9p)^7 (0.9q) + {8 \choose 2} (0.9p)^6 (0.9q)^2 + \ldots + {8 \choose 8} (0.9p)^0 (0.9q)^8$

Observe that this is a binomial expansion.

Required probability $= (0.9p + 0.9q)^8 = 0.43046721$ (exact)

(i)
$\text{P}(A' \cap B') = 1 - \text{P}(A \cup B)$

$\text{P}(A' \cap B') = 1 - [\text{P}(A) + \text{P}(B) - \text{P}(A \cap B)]$

$\text{P}(A' \cap B') = 1 - a - b + ab$

$\text{P}(A' \cap B') = (1-a) - b(1-a)$

$\text{P}(A' \cap B') = (1-b)(1-a)$

$\text{P}(A' \cap B') = \text{P}(B') \times \text{P}(A')$

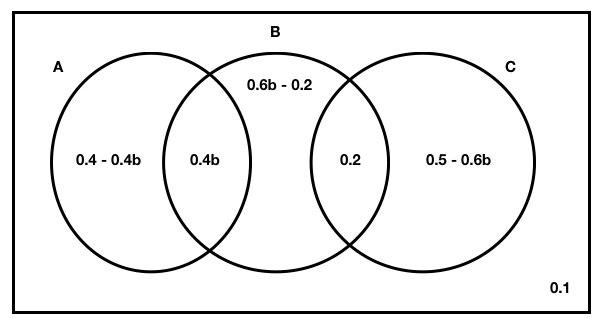
Thus, events $A'$ and $B'$ are independent events.

(ii)
$\text{P}(A' \cap C') = 1 - \text{P}(A \cup C)$

$\text{P}(A' \cap C') = 1 - [\text{P}(A) + \text{P}(C) - \text{P}(A \cap C)]$

$\text{P}(A' \cap C') = 1 - a - c$

If $A'$ and $C'$ are mutually exclusive, then $\text{P}(A' \cap C') = 0$ .

$1 - a - c = 0 \Rightarrow a + c = 1$

Venn Diagram for 7ii

Note: Students can draw different “designs” so long as you show that A and C does not intersect and they fill up the entire venn diagram.
(iii)
Since $A$ and $B$ are independent, $\text{P}(A \cap B) = ab = \frac{2}{5}b = 0.4b$ , $\text{P}(B \cap C) = 0.2$ . We can find the following information.

Venn Diagram for 7iii

Suppose $0.5 - 0.6b = 0 \Rightarrow b = 0.5$ , then $\text{P}(A \cap B) = 0.4b = \frac{1}{3}$

Suppose $0.5 - 0.6b = 1 - 0.4 - 0.1 - 0.2 \Rightarrow b = \frac{1}{3}$ , then $\text{P}(A \cap B) = 0.4b = \frac{2}{15}$

Minimum $\text{P}(A \cap B) = \frac{2}{15}$

Maximum $\text{P}(A \cap B) = \frac{1}{3}$

(i)

Probability Distribution for S

$\text{P}(S = 6 ) = \frac{2}{(n+5)(n+4)}$

$\text{P}(S = 7 ) = \frac{12}{(n+5)(n+4)}$

$\text{P}(S = 8 ) = \frac{4n+6}{(n+5)(n+4)}$

$\text{P}(S = 9 ) = \frac{6n}{(n+5)(n+4)}$

$\text{P}(S = 10 ) = \frac{n(n-1)}{(n+5)(n+4)}$

(ii)
When $n = 1, \text{P}(S = 10 ) = 0$ .

In order to have $S =10$ , two balls numbered 5 needs to be drawn which is not possible since balls are taken without replacement and there is only one ball numbered 5.

(iii)
$\mathbb{E}(S) = \frac{1}{(n+5)(n+4)}[6(2) + 7(12) + 8(4n + 6) + 9(6n) + 10(n)(n-1)]$

$\mathbb{E}(S) = \frac{1}{(n+5)(n+4)}(144 + 76n + 10n^2)$

$\mathbb{E}(S) = \frac{1}{(n+5)(n+4)}(10n + 36)(n+4)$

$\mathbb{E}(S) = \frac{10n + 36}{n+5}$

$\mathbb{E}(S^2) = \frac{1}{(n+5)(n+4)}[6^2(2) + 7^2(12) + 8^2(4n + 6) + 9^2(6n) + 10^2(n)(n-1)]$

$\mathbb{E}(S^2) = \frac{1}{(n+5)(n+4)}[1044 + 642n + 100n^2]$

$\text{Var}(S) = \mathbb{E}(S^2) - \mathbb{E}(S)^2$

$\text{Var}(S) = \frac{1}{(n+5)(n+4)}[1044 + 642n + 100n^2] - (\frac{10n + 36}{n+5})^2$

$\text{Var}(S) = \frac{1}{(n+5)^2(n+4)}[(1044 + 642n + 100n^2)(n+5) - (100n^2 + 720n + 1296)(n+4)]$

$\text{Var}(S) = \frac{1}{(n+5)^2(n+4)}[1044n + 642n^2 + 100n^3 + 5220 + 3210n + 500n^2 - (100n^3 + 720n^2 + 1296n + 400n^2 + 2880n + 5184)]$

$\text{Var}(S) = \frac{1}{(n+5)^2(n+4)}(36 + 22n^2 + 78n)$

$\therefore g(n) = 22n^2 + 78n + 36$

(i)

Scatter Diagram for 9i

The relationships is unlikely to be well modelled by the equation $P = aR + b$ . From the scatter, we observe that as $R$ increases, $P$ increases at an increasing rate. Thus, a linear model such as $P = aR + b$ would not be suitable.
(ii)
r value for $P = aR + b = 0.96929 \approx 0.969$ (3 SF)

r value for $P = aR^2 + b = 0.99303 \approx 0.993$ (3 SF)

Since the r value for $P = aR^2 + b$ is close to 1, $P = aR^2 + b$ is a better model for the relationship between $P$ and $R$ .

$P = 2.8457 \times 10^{-8} R^2 - 0.2826076$

$P = 2.85 \times 10^{-8} R^2 - 0.283$ (3 SF)

(iii)
$P = 0.9, R = 6446 \approx 6450$ revolutions per minute.

Thus estimate is reliable as $P = 0.9$ is within the given date range and interpolation is a good practice.

(iv)
$R = 3300, P = 0.0272939 \approx 0.0273$ watts

Thus estimate is not reliable as $R = 3300$ is not within the given date range and extrapolation is a bad practice as it assumes the trend continues outside of the data range.

(v)
Replace $R$ by $60R$ .

$P = 2.8457 \times 10^{-8} (60R)^2 - 0.2826076$

$P =1.02 \times 10^{-4} R^2 - 0.283$ (3 SF)

Q10

(i)

Graph of P(40 < T < 60)

(ii)
Let $X$ denote the mass of one type of light bulb, in grams.

$X \sim \text{N}(50, 1.5^2)$

$\text{P}(X \textless 50.4) = 0.605$ (3 SF)

(iii)
Let $Y$ denote the mass of of an empty box, in grams.

$Y \sim \text{N}(75, 2^2)$

$Y_1 + \ldots + Y_4 \sim \text{N}(300, 16)$

$\text{P}(Y_1 + \ldots + Y_4 \textless 50.4) = 0.773$ (3 SF)

(iv)
$X + Y \sim \text{N}(126, 6.25)$

$\text{P}(124.9 \textless X + Y \textless 125.7) = 0.126$ (3 SF)

(v)

$Y + X_1 + 0.3X_2 \sim \text{N}(140, 6.4525)$

$\text{P}(Y + X_1 + 0.3X_2 > k) = 0.9$

Using GC, $k = 136.74 \approx 137$ (3 SF)

(vi)
Let $T$ denote the mass of a randomly chosen box, each containing a bulb and padding in grams.

$T = Y + X_1 + 0.3X_2 \sim \text{N}(140, 6.4525)$

$T_1 + \ldots + T_4 \sim \text{N}(560, 25.81)$

$\text{P}(T_1 + \ldots + T_4 > 565) = 0.163$ (3 SF)

Relevant materials

MF26

KS Comments

2018 A-level H2 Mathematics (9758) Paper 2 Suggested Solutions2018-08-232022-09-05http://theculture.sg/wp-content/uploads/2018/03/the-culture-logo.pngThe Culture SGhttp://theculture.sg/wp-content/uploads/2018/03/the-culture-logo.png200px200px

About

KS Teng

KS has been teaching H2/H1 Mathematics and IB mathematics for the more than 10 years. Having taught students from all various Junior Colleges, KS adapts to students' abilities and help them better understand the topics. As someone who loves to teach mathematics and sees it as a truly useful tool in life, KS seeks to enable students to appreciate math. Therefore, his tuition mission is to motivate and cultivate students to be independent and confident thinkers.

Comments

siva November 10, 2022
Reply
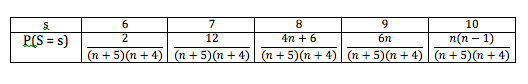
hi i think 9v is wrong as question states R as no of revolutions per minute and as such we must substitute r with r/60 instead please correct me if im wrong

Cart

2018 A-level H2 Mathematics (9758) Paper 2 Suggested Solutions

Relevant materials

KS Comments

Leave a Comment
Cancel reply

2018 A-level H2 Mathematics (9758) Paper 2 Suggested Solutions

Relevant materials

KS Comments

Leave a Comment Cancel reply

Leave a Comment
Cancel reply