Hopefully, you guys have started on the Set A. You will find the following solutions useful. Click on the question. Please do attempt them during this December Holidays. 🙂
If you do have any questions, please WhatsApp me. 🙂
Firstly, either consider or square both sides. Its easier to do the latter (so I will).
Since for all
(i)
LHS
RHS.
(ii)
(Showing workings for Method of Difference)
(iii)
As .
Hence, the series converges to .
(i)
Let
— (1)
— (2)
— (3)
Using GC,
(ii)
Since arithmetic series is increasing, ,
Common ratio
Since , the geometric series converges.
(ii)
First term
By Pythagoras’ Theorem,
since
Area,
Let
Check with first derivative test.
Hence, maximises A.
Thus, dimensions are m and
m (to 3.s.f.).
(i)
, where
is an arbitrary constant.
When .
(ii)
As .
Thus, the population of salmon in the fish farm will decrease towards 6,000 in the long run.
(a)
(b)
(c)
For curve to cut the x axis at 2 distinct points,
or
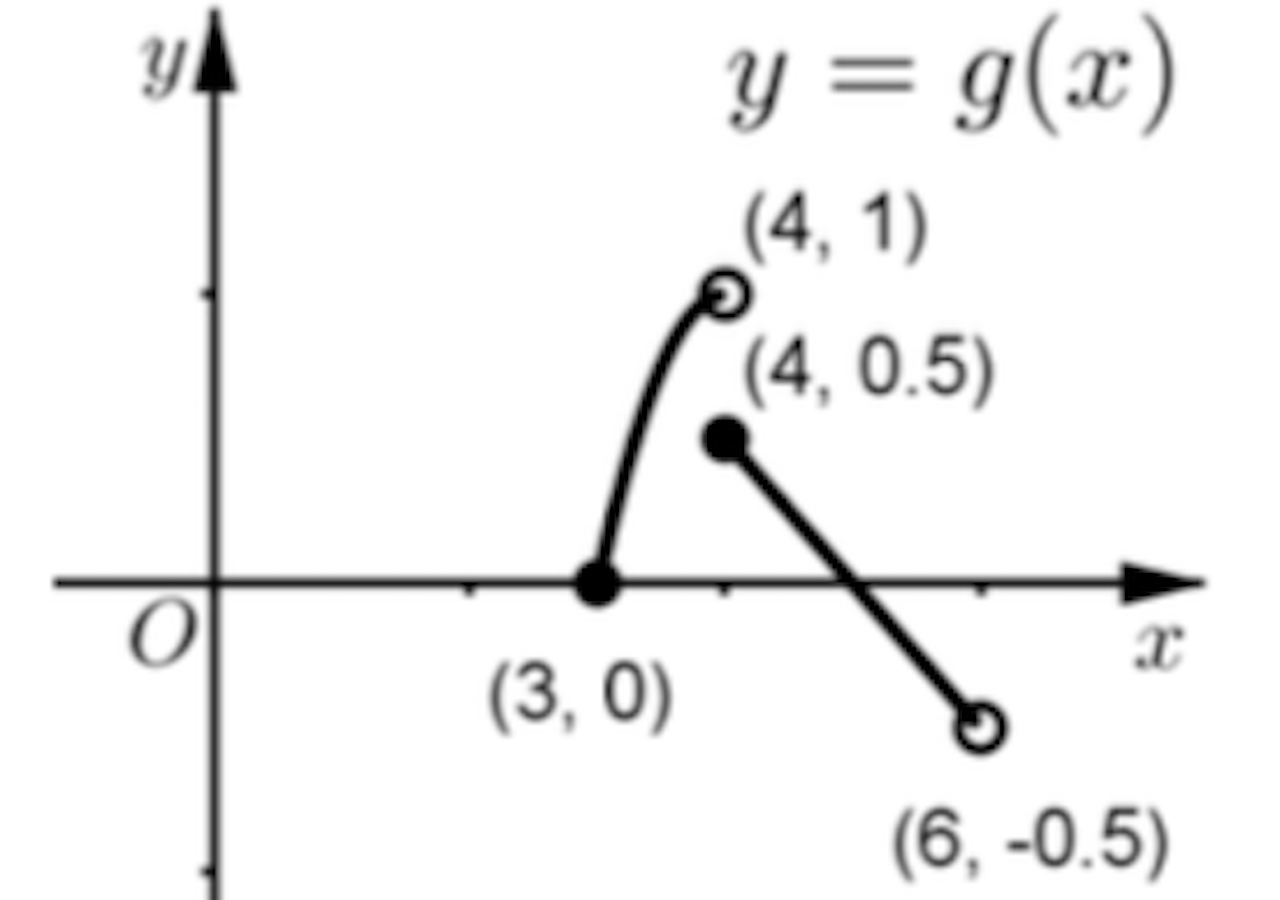
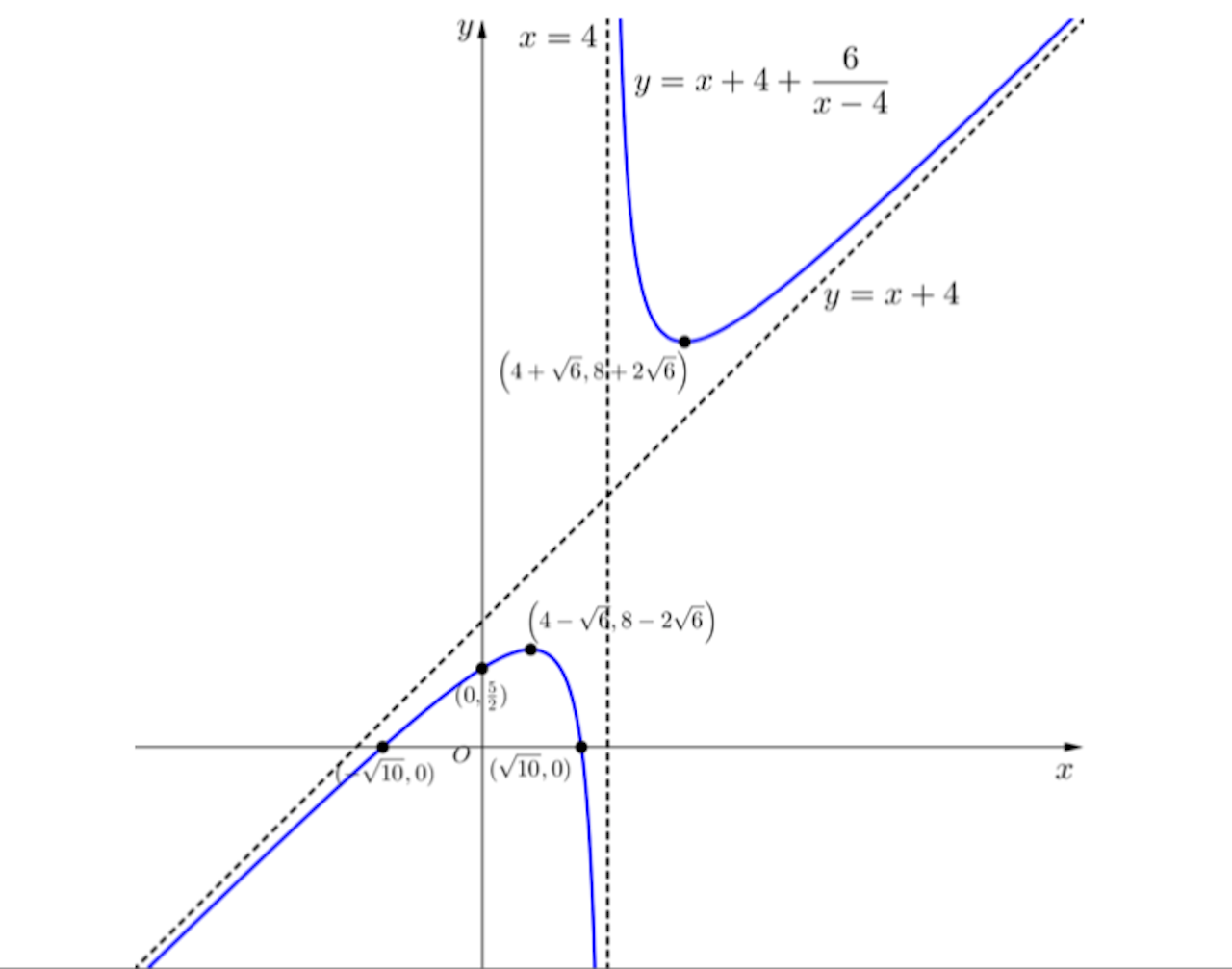
(i)
(ii)
— (1)
— (2)
Coordinate of
When
Observe that passes through
for all real values of
.
Thus, for line to not intersect C, .
(iii)
or
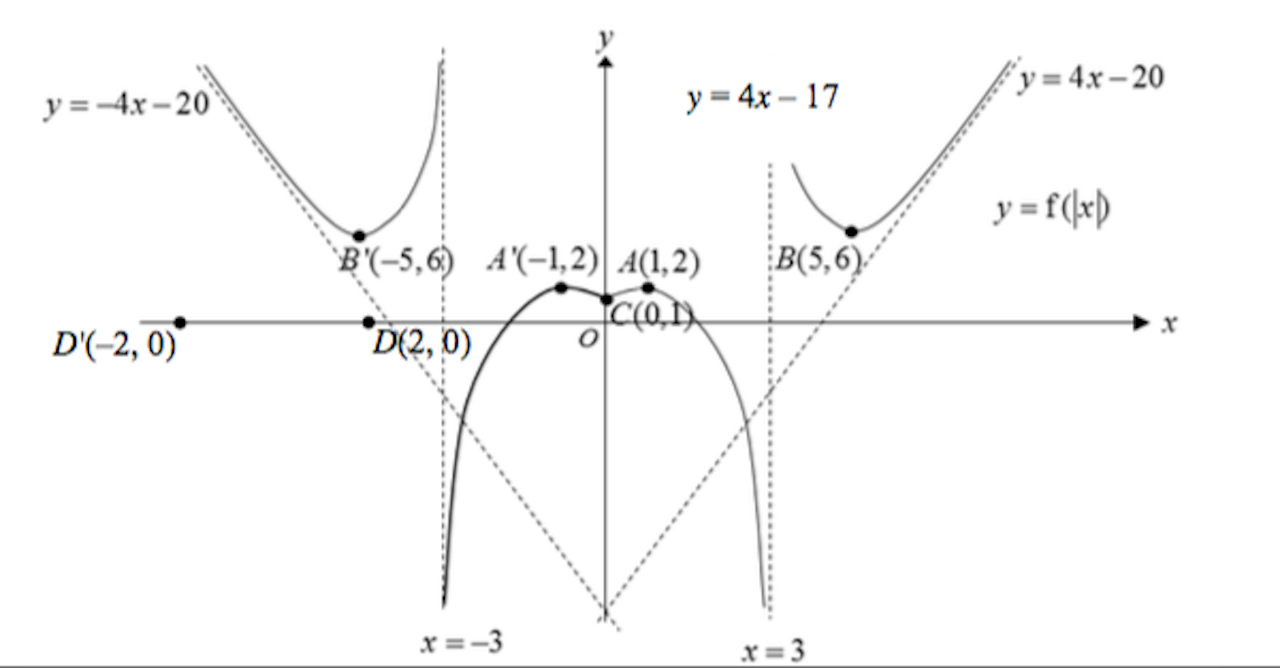
(a)
(b)
(i)
(ii)
When
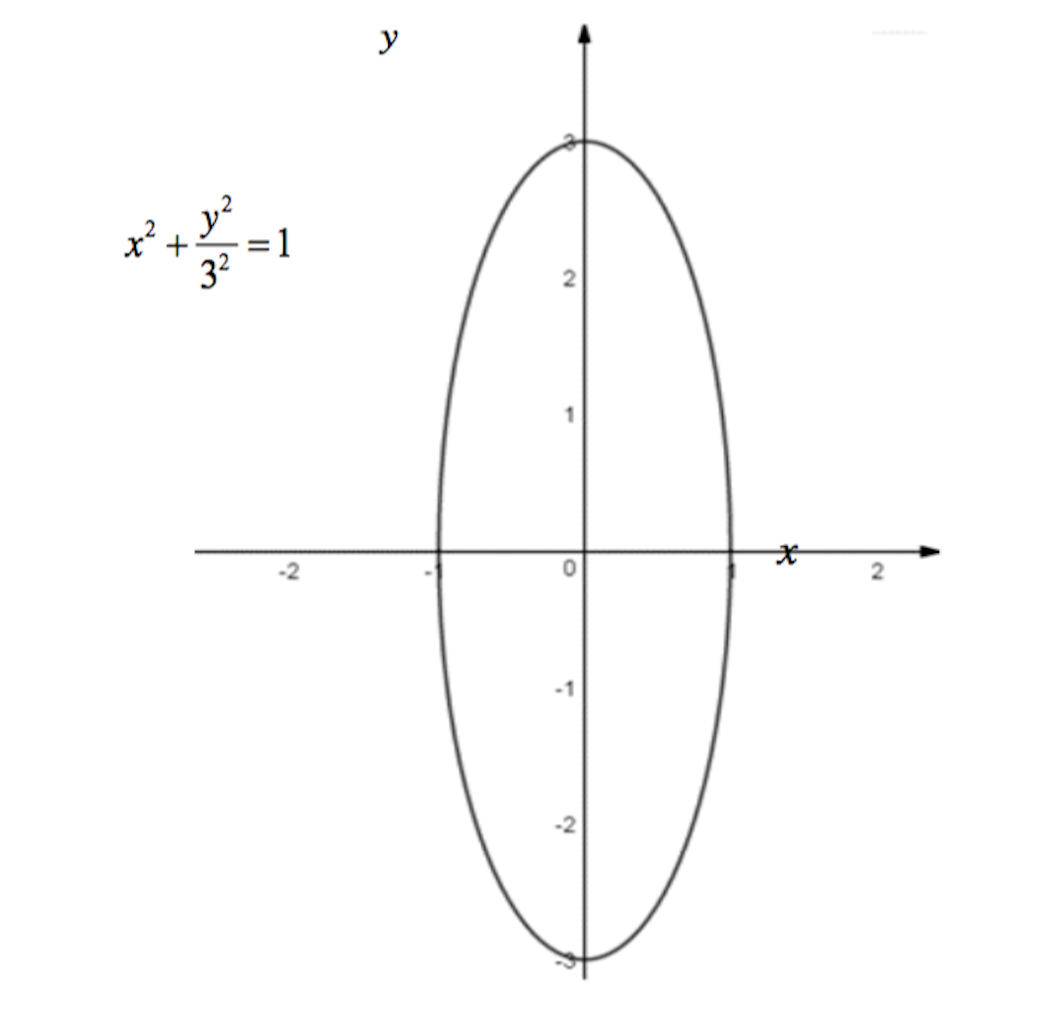
(a)
Firstly, scale by factor parallel to the y – axis.
Secondly, translate by 3 units in the positive x – direction.
Curve D is a circle.
(b)
(i)
(ii)
(i)
Since
Using Sine Rule,
So y coordinate is given by
(ii)
Gradient of
Since QS is always tangential to hump, gradient of
Since
(iii)
Area
(4dp)
Relevant Materials: MF26