2024 A-level H2 Mathematics (9758) Paper 2 Suggested Solutions

All solutions here are suggested. Mr. Teng will hold no liability for any errors. Comments are entirely personal opinions
Numerical Answers (workings/explanations are after the numerical answers. Click to expand.)

Question 1: $a = -1, b = -4; 2+6i$
Question 2: $23.43 \text{m}^2$
Question 3: $(-\infty, 11]; 7$
Question 4: $t_n = 6n^2 - 22n + 6; n = 5 \text{~or~} 7; 196$
Question 5: $y=3$
Question 6: Sample; $\frac{13}{32}$
Question 7: $\frac{2}{n}; n \ge 37, n \in \mathbb{Z}^+; \frac{1}{14}$
Question 8: $\alpha = 4$
Question 9: $\frac{1}{80}; 0.298; 8.36; 0.506$
Question 10: $0.941; 0.183; 0.769; 6377$
Question 11: $d=33.0, e=17.3, r= 0.991; 510; 26.75; -1.2 < c < 1$

Total marks: 6 marks

(a)
$-2 + i = a(-2 - i) + b$
By comparing real and imaginary parts,
$1 = -a \Rightarrow a = -1$
$-2 = -2a + b \Rightarrow b = -4$

(b)
$(1-3i)(-2+i) - \frac{1-3i}{-2+i}$
$= -2 + i + 6i + 3 - \frac{(1-3i)(-2-i)}{(-2)^2 + i^2}$
$= 1 + 7i - \frac{-2-i + 6i - 3}{5}$
$= 1 + 7i + 1 - i$
$= 2 + 6i$

Total marks: 6 marks

Perimeter $= 2b + 3 a = 20 \Rightarrow b = 10 - 1.5a$
Area, $A = ab + \frac{1}{2}(a^2)\sin 60^{\circ}$
$A = a (10 - 1.5a) + \frac{\sqrt{3}}{4} a^2$

The equation did not specify to use “calculus”, we may solve this graphically.

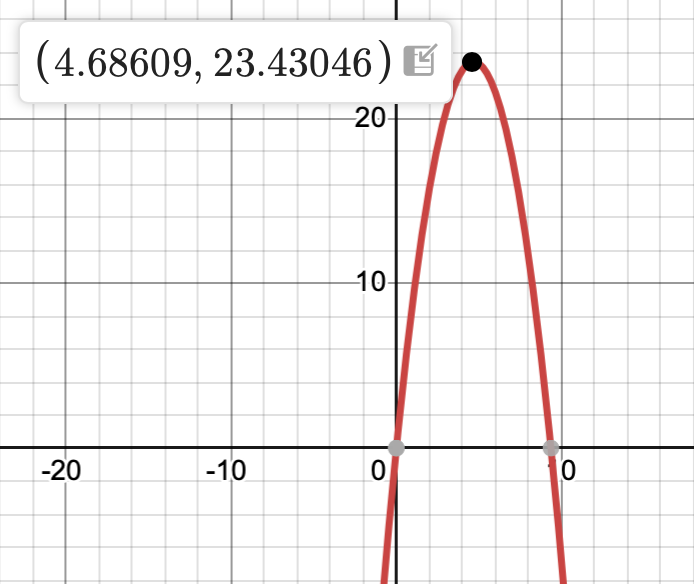
From the graph, the maximum point occurs when $a = 4.68609$ and the maximum area $\approx 23.43 \text{m}^2$

There is also discussion regarding the question phrasing, attached below.

And it seems that $a$ should be the shorter side, which suggests that $a < b$ , now if that is the case, we will have that $a < b = 10 - 1.5a \Rightarrow a < 4$ . Should $a < 4$ , then the maximum area is $22.92 \text{m}^2$ . I do not know which is the better one. So we will only find out in 2025.

Total marks: 8 marks

(a)
$f(x) = - (x - 2)^2 + 11$
$\Rightarrow R_f = (- \infty , 11]$

(b)

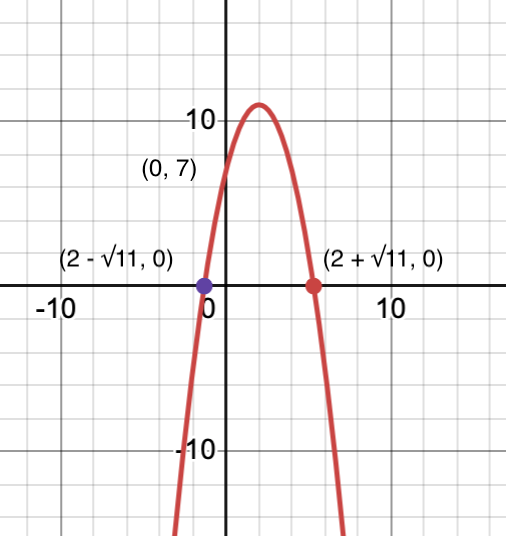
(c)
Method 1:
Draw the graph of $g(x)$ , do label the endpoints and explain the horizontal line test, i.e., Any horizontal line $y=k$ cuts the graph of $g$ at most once, thus, $g$ is a one-one function and $g^{-1}$ exists.

Method 2:
$g'(x) = - \frac{2}{x^2}$
For $1 \le x \le 10$ , we have the $x^2 >0$ and $g'(x) < 0$ , thus $g$ is a strictly decreasing function and thus it is one-one. Thus, $g^{-1}$ exists.

(d)
$g^{-1}(x) = \frac{2}{x-1}$
$fg^{-1}(1.5) = f( \frac{2}{1.5-1}) = f(4) = 7$

Total marks: 9 marks

(a)
$t_n = T_n - T_{n-1}$
$t_n = 2n^3 - 8n^2 - 4n - [2(n-1)^3 - 8(n-1)^2 - 4(n-1)]$
$t_n = 2n^3 - 8n^2 - 4n - [2(n^3 - 3n^2 + 3n-1) - 8(n^2 - 2n +1) - 4(n-1)]$
$t_n = 6n^2 - 22n + 6$

(b)
$50n - 204 = 6n^2 - 22n + 6$
$6n^2 - 72 n + 210 = 0$
Using GC, $n= 5$ or $n= 7$

(c)
Using GC,
When $n = 8, u_n = 196$
When $n = 60, v_n = 196$
Thus, the smallest number greater than 100 in both series U and series V is $196$ .

(d)(i)
$w_n = 3n^2 - 4n + 7$
$w_n = 3n(n - 1) - 2n +7$
Since $n$ may be an odd or even number, observe that if $n$ is odd, then $(n-1)$ is even. Similarly if $n$ is even, then $(n-1)$ is odd. Clearly, the product of an odd and even number is even. This implies that $3n(n-1)$ is an even number.
Since $2n$ is a multiple of two, it must be even. $7$ is an odd number.
The sum of two even number is an even number, then $3n(n - 1) - 2n$ is an even number.
The sum of an even and an odd number is an odd number, then $3n(n - 1) - 2n +7$ must be an odd number. Thus all terms in series $W$ are odd numbers.

(d)(ii)
$u_n = 50n - 204 = 2(25n - 102)$ .
Since $u_n$ is a multiple of two, all terms in series $U$ are even numbers.
Since all terms in series $W$ are odd numbers, then series $U$ and series $W$ do not have any terms in common.

Total marks: 11 marks

(a)(i) Reflection of $y=f(x)$ about (in) the $y=x$ line

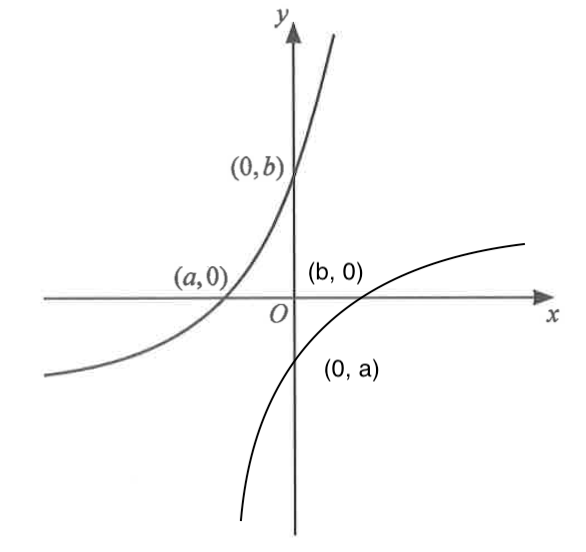
(a)(ii) Using the above graph, translate 1 units in positive $x$ direction and scale by factor 2 parallel to the $y$ axis. Note question does not require the $y$ -intercept, which we should not be able to give.

(b)(i)

(b)(ii)

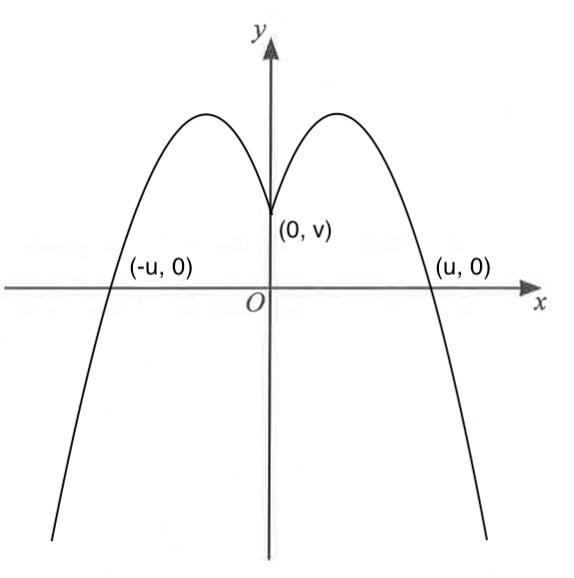
(c)(i)(ii)

(c)(iii) The lines of symmetry is $y = 3$

Total marks: 6 marks

(a) The 65 member comprise a sample since they form a subset of the population of 90 members.

(b) A random sample ensures that each member has an equal chance of being selected, independently of each other. This will avoid bias.

(c)
We start by finding the total number of ways:
AAAYYS: $\binom{45}{3}\times \binom{27}{2} \times \binom{18}{1} = 89652420$
AAAYSS: $\binom{45}{3}\times \binom{27}{1} \times \binom{18}{2} = 58618890$
AAAAYS: $\binom{45}{4}\times \binom{27}{1} \times \binom{18}{1} = 72411570$
Required probability $= \frac{89652420}{89652420 + 58618890 + 72411570} = \frac{13}{32}$

Total marks: 8 marks

(a)(i)
Required probability $= \frac{1}{n} \times \frac{n-1}{n-1} \times 2 = \frac{2}{n}$

(a)(ii)
Required probability $= \frac{n-1}{n} \times \frac{n-2}{n-1} \times \frac{1}{n-2} \times \frac{n-3}{n-3} \times 2 = \frac{2}{n}$

(a)(iii)
$1 - \frac{2}{n} - \frac{2}{n} > 8 (\frac{2}{n} + \frac{2}{n})$
$n > 36$
$n \ge 37, n \in \mathbb{Z}^+$

Total marks: 8 marks

(a) Lee should carry out a one-tailed test since he is testing for a definite decrease (reduction)

(b) Let $\mu$ be the population mean number of birds visiting the feeder every morning.
$H_0: \mu = 17.3$
$H_1: \my < 17.3$

(c)
Unbiased estimate of population mean $= \frac{512}{32} = 16$
Unbiased estimate of population variance $= \frac{1}{32-1} (8702 - \frac{512^2}{32}) = \frac{510}{31}$

Under $H_0, \bar{X} \sim \text{N}(17.3, \frac{ \frac{510}{31}}{32})$ approximately by central limit theorem since sample size, $n = 32 \ge 30$ is sufficiently large.
Using GC, p-value $= \text{P}( \bar{X} < 16) = 0.034910$
For $H_0$ to be rejected, p-value $\le \frac{\alpha}{100} \Rightarrow \alpha \ge 3.4910$
Thus, the minimum possible integer value of $\alpha$ is $4$ .

(d) Lee can conclude with sufficient evidence at $4\%$ level of significance that the mean number of birds visiting the feed in 20 minutes each morning has reduced.

(e) Since the distribution for the number of birds visiting the feeder every morning is unknown, a sample size of 10 mornings will be not sufficiently large to approximate the sample mean distribution to a normal distribution by central limit theorem.

Total marks: 11 marks

(a) Credits: https://people.hsc.edu/faculty-staff/blins/StatsTools/binomialPlotter.html

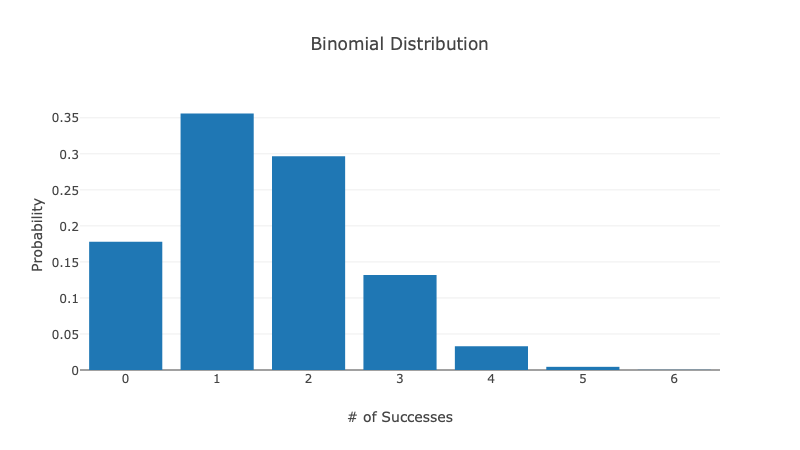
(b) Required probability $= \frac{9 \times 1 + 6 \times 2 + 4 \times 3 + 3 \times 4 + 1 \times 5}{4000} = \frac{1}{80}$

(c)(i) The event that a randomly chosen burger has insufficient cheese in them is independent of another randomly chosen burger having insufficient cheese in them. The probability of a randomly chosen burger having insufficient cheese in them is constant at $\frac{1}{80}$ for each trial.

(c)(ii)
$Y \sim \text{B} (120, 0.03)$
Required probability
$= \text{P}( Y < 3)$
$= \text{P}( Y \le 2)$
$\approx 0.298$

(c)(iii) Required answer $= 28 \times 0.298435 \approx 8.36$ (3 s.f.)

(c)(iv) Let $W$ be the number of burgers found to have insufficient cheese in them, out of 3360 burgers.
$W \sim \text{B}(3360, 0.03)$
Required probability
$= \text{P}(W > 100)$
$= 1 - \text{P}(W \le 100)$
$\approx 0.506$

Q10

Total marks: 11 marks

(a) Let $X$ be the masses of plates before drilling, in grams.
$X \sim \text{N}(200, 1.6^2)$
Required probability
$= \text{P}(X > 197.5)$
$\approx 0.941$

(b) $0.95X \sim \text{N}( 190, 2.3104)$
$\text{P}(190 < 0.95X < 192) = 0. 40587$
Let $Y$ be the number of plates with masses between 190g and 192g, out of 8 plates.
$Y \sim \text{B}(8, 0.40587)$
Required probability
$= \text{P}(Y \ge 5)$
$= 1 - \text{P}(Y \le 4)$
$\approx 0.183$

(c) Let $R = 0.95X_1 + \ldots 0.95X_{20}$
$R \sim \text{N}(3800, 46.208)$
Required probability
$= \text{P}(R < 3805)$
$\approx 0.769$

(d) Let $S$ and $B$ be the masses of screws and bolts respectively, in grams.
$S \sim \text{N}(10, 0.3^2)$
$B \sim \text{N}(44, 1.1^2)$
For 20 drilled plates, we need 80 screws and 40 bolts.
Let $T = R + S_1 + \ldots + S_{80} + B_1 + \ldots + B_{40}$
$T \sim \text{N}(6360, 101.808)$
Let $t$ be the mass exceeded by just $5\%$ of the value packs.
$\text{P}(T > t) = 0.05$
$\Rightarrow t = 6376.59 \approx 6377$

Q11

Total marks:

(a)(i) The scatter diagram suggests that as $m$ increases, $p$ increases decreasingly.

(a)(ii) Observe that both given models suggest the same trend and we will need to calculate the product moment correlation coefficient (PMCC) to select the appropriate model.
Using GC, PMCC for $p = a + b \ln m = 0.92851$
Using GC, PMCC for $p = d + e \sqrt{m} = 0.99143$
Since the PMCC for $p = d + e \sqrt{m}$ is close to 1, it suggests stronger positive linear correlation between $p$ and $\sqrt{m}$ . Thus, the more appropriate model. Using GC, we find that $d=33.0, e=17.3$ and PMCC $= 0.991$

(a)(iii) $p = 32.952 + 17.269 \sqrt{750} \approx 510$ cents.
Since $750$ is within the data range of $24 \le m \le 5000$ , this is interpolation and the estimate is reliable.

(b)(i)

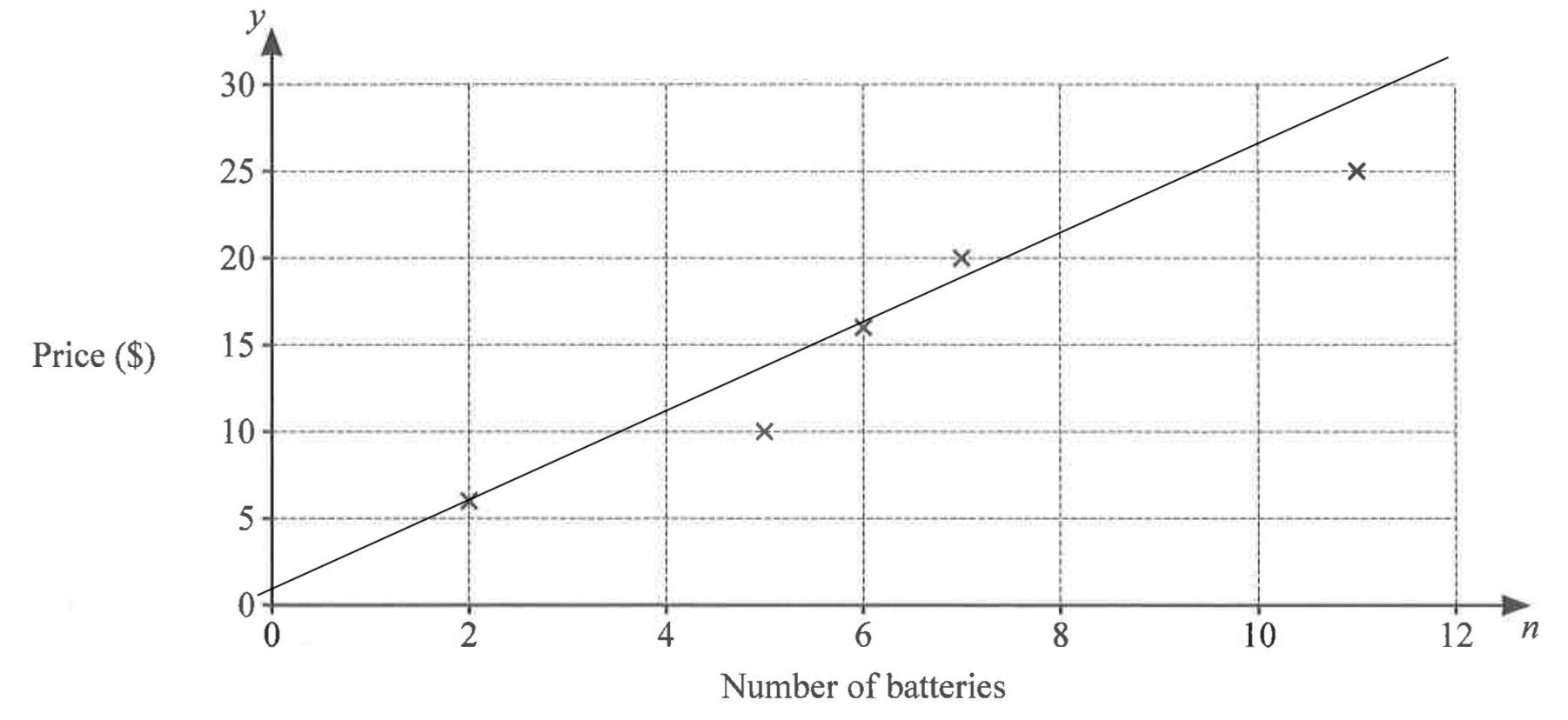
(b)(ii)(A)

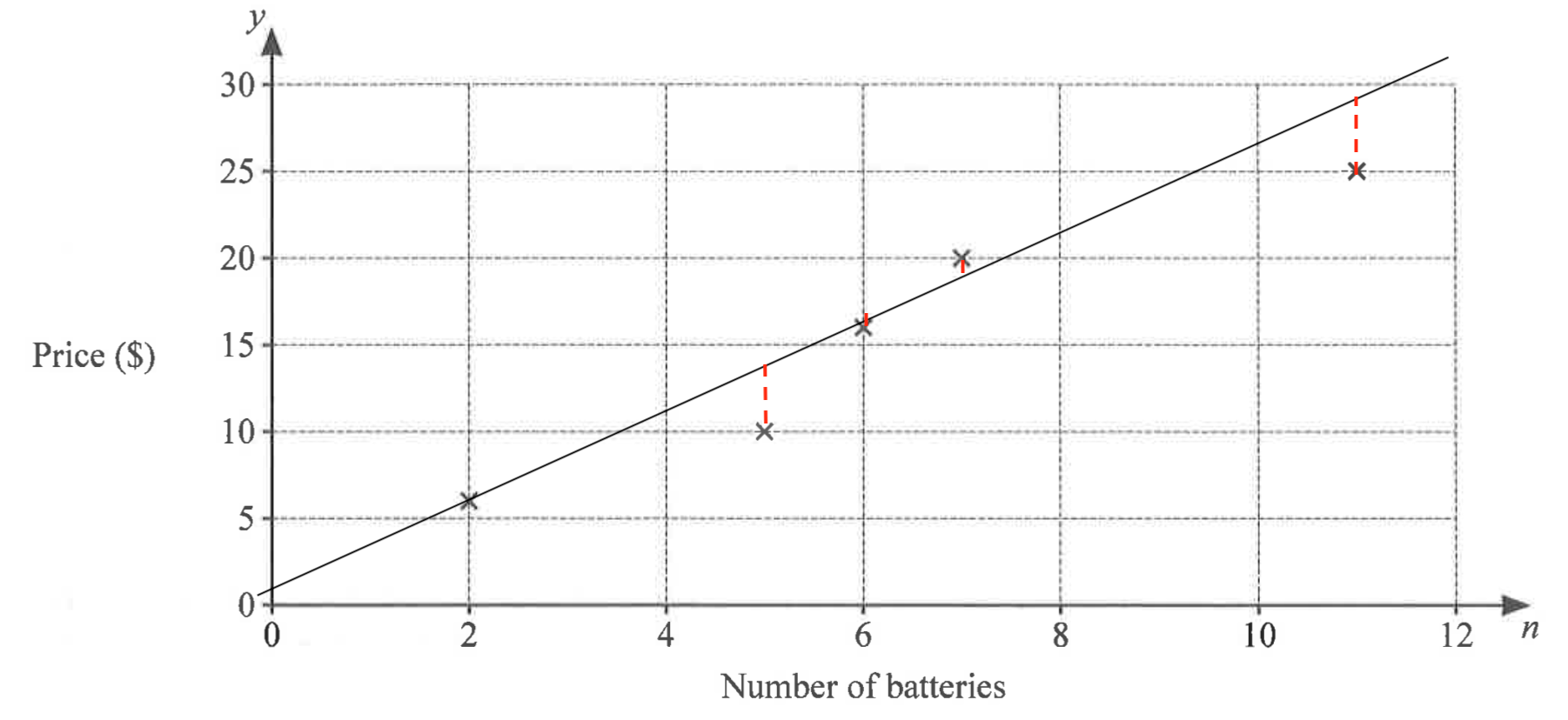
(b)(ii)(B) The residuals may be either positive or negative, and adding them out may result in them cancelling each other out. Squaring the residuals instead ensure the values will be positive and adding these values up will give a more meaningful result.

(b)(ii)(C) Using GC, required answers $= 26.75$

(b)(iii) The sum of squares of the residuals will have to be of a smaller value.

(b)(iv)
Sum of squares of residuals
$= (1 - c)^2 + (-2.5 - c)^2 + (1-c)^2 + (2.5 - c)^2 + (-2.5-c)^2$
$= 5c^2 + c + 20.75$
Let $5c^2 + c + 20.75 < 26.75$
We have that $-1.2 < c < 1$

2024 A-level H2 Mathematics (9758) Paper 2 Suggested Solutions2024-10-172024-11-09http://theculture.sg/wp-content/uploads/2018/03/the-culture-logo.pngThe Culture SGhttp://theculture.sg/wp-content/uploads/2018/03/the-culture-logo.png200px200px

About

KS Teng

KS has been teaching H2/H1 Mathematics and IB mathematics for the more than 10 years. Having taught students from all various Junior Colleges, KS adapts to students' abilities and help them better understand the topics. As someone who loves to teach mathematics and sees it as a truly useful tool in life, KS seeks to enable students to appreciate math. Therefore, his tuition mission is to motivate and cultivate students to be independent and confident thinkers.

Cart

2024 A-level H2 Mathematics (9758) Paper 2 Suggested Solutions

Leave a Comment
Cancel reply

2024 A-level H2 Mathematics (9758) Paper 2 Suggested Solutions

Leave a Comment Cancel reply

Leave a Comment
Cancel reply