All solutions here are suggested. Mr. Teng will hold no liability for any errors. Comments are entirely personal opinions
Numerical Answers (workings/explanations are after the numerical answers. Click to expand.)
Question 1:
Question 2:
Question 3:
Question 4: or
;
Question 5:
Question 6:
Question 7:
Question 8:
Question 9:
Question 10:
Question 11:
Question 12: ; cannot see since
;
; no
Total marks: 4 marks
Since
At
At
Using GC,
Note: Students can verify this answer by plotting the graph.
Total marks: 3 marks
Since is a unit vector, then
.
Since , then
, where
is the angle between
and
Thus, and
are perpendicular to each other.
Total marks: 4 marks
(a) For to be a root, we must be able to apply conjugate root theorem. Thus, all coefficients of the polynomial should be real numbers. We have that
.
(b)
Thus,
Note: Students can verify this answer by using the GC’s polynomial root finder function
Total marks: 6 marks
(a)
or
(b)
Replace by
(rejected,
or
Note: Students can verify this answer by plotting the graph.
Total marks: 7 marks
(a)
(b) Using GC, since ,
and
(c) Note that is a GP with first term
and common ratio
.
Total marks: 8 marks
(a)
When
Tangent at is given by
Thus,
(b) Since the tangent cuts the origin, then
(c)
Required area, using GC.
Total marks: 8 marks
(a)
(b) Note: there are some issues with the rendering here, you should do method of difference.
(c)
Observe that .
Thus, the possible values are
Note: Students can verify this answer by calculating the sigma with the GC.
Total marks: 9 marks
(a)
Thus,
(b)
When
Thus,
Note: we can verify the answers using standard series in exam.
(c)
By comparing, and
.
Total marks: 10 marks
(a), and
(b)
Let , then
Thus, coordinates are .
(c) Observe that when
Total marks: 14 marks
(a)
(b) The axial intercepts are , and the asymptotes are
and
.
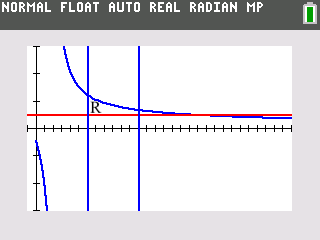
(c)
Thus,
(d) using GC.
Total marks: 12 marks
(a)(i)
Thus,
(a)(ii) We have that
(b), where
Let
Thus, and
(c)
When
Let , using GC,
seconds
Total marks: 15 marks
(a) Let be the points nearest to the controller and let
be the controller.
for some
Thus, the coordinates of is
.
(b)
Thus, the controller will not be able to see.
(c) (i) Clearly, the paths are not parallel. Now suppose the path intersect, then
We have that
Solving, and
. Thus
.
Thus,
(c) (ii)
Required angle
(d) (i)
(d) (ii)
For the distance to be the shortest, the resultant vector should be perpendicular to both the paths.
Since the resultant vector is not perpendicular to both the paths, the distance found is not the shortest.

Hi, for 11(b) B should be -2 if I remember correctly? Because in the question, exponent for e^Bt had no negative sign in front of the B.
I also need to verify if Q9(c) definite integral range is from 0 to Q or P to Q. Because I may have misread the question during the paper but I did from P to Q.
I’m not sure if you have access to the paper exactly as it is, in that case, my information could be wrong.
Hello, actually I can’t recall whether they had a negative sign in from of the exponent too. For 9c, I think it was 0 to Q, but I may have recalled wrongly too.
Hello, for qn 6 (c), was the answer supposed to be in 1dp/2sf? I recall giving mine to 3sf as 5.40
hello. its to 1 decimal places.