All solutions here are suggested. Mr. Teng will hold no liability for any errors. Comments are entirely personal opinions. Sorry, due to copyrights issues, I cannot share the paper.
Numerical Answers (workings/explanations are after the numerical answers.)
Question 1:
Question 2:
Question 3:
Question 4:
Question 5: ; refer to solutions below for explanation.
Question 6:
Question 7:
Question 8:
Question 9:
Question 10:
Question 11:
(i) [Maximum marks: 2]
(ii) [Maximum marks: 2]
Required angle
Note/ Comment: For (i), if students simplified, it is ok since the questions simply asked for a vector normal to the plane.
Differentiating with respect to ,
Let
, where
Note/ Comment: Slightly tedious but fully manageable. A more elegant approach will be to consider
and differentiate from here.
(i) [Maximum marks: 3]
(ii) [Maximum marks: 4]
Note/ Comment: Quite a standard question. Students can verify their answers using the standard series too.
(i) [Maximum marks: 4]
We can first convert all the given complex numbers to exponential form.
*Another approach is to straightaway solve for and
.
(ii) [Maximum marks: 3]
, and
* Another approach is to observe that
Note/ Comment: This can be tedious if the student did not use exponential form to get around things. For (ii), the question has stated “possible values” which is a hint that there is more than one answer to be expected. Aside from it, students should use GC to check their answers.
(i) [Maximum marks: 2]
We have that , so
is parallel to
and
where
is a scalar.
(ii) [Maximum marks: 3]
R is a set of points lying on a line that passes through position vector and is parallel to direction vector
, where
is a scalar parameter.
(iii) [Maximum marks: 4]
R is a set of point lying on a plane that passes through position vector and is perpendicular to normal vector
, where
units is the shortest displacement R is from the origin.
Note/ Comment: Quite a standard question, from the specimen paper and also from 2017 A’levels paper. Students should be able to answer this comfortably.
By comparing the coefficients,
Other root and
and
Note/ Comment: Actually this question did not prohibit the GC, so the last part could be done easily.
(i) [Maximum marks: 1]
(ii) [Maximum marks: 4]
(iii) [Maximum marks: 4]
Note/ Comment: Students should use GC to check their answers.
(a)(i) [Maximum marks: 2]
(a)(ii) [Maximum marks: 3]
(b)(i) [Maximum marks: 2]
(b)(ii) [Maximum marks: 5]
Smallest possible .
Note/ Comment: Standard question.
(i) [Maximum marks: 1]
Consider the lines and
, since the gradient
, where
is the angle the line makes with the horizontal and the two lines
and
are perpendicular, i.e.,
radians spaced from each other. We have that
.
(ii) [Maximum marks: 3]
Since , required set
(iii) [Maximum marks: 3]
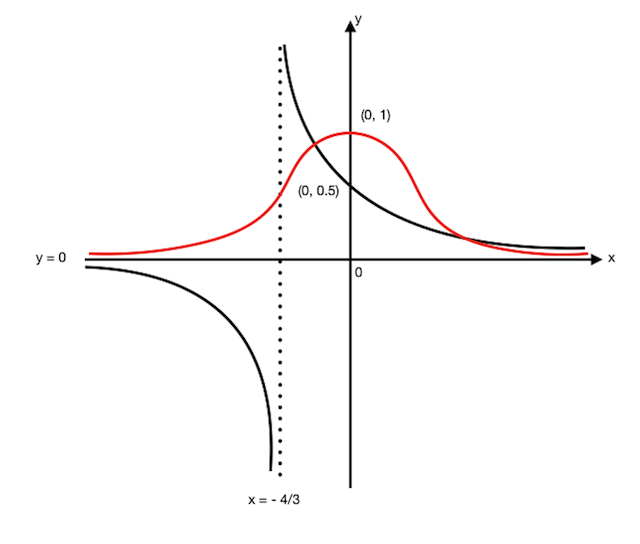
Note: Right side of the graph. The asymptote is y=0, I do not know why wordpress cut it off. 🙁
(iv) [Maximum marks: 5]
Using GC,
(i) [Maximum marks: 2]
(ii) [Maximum marks: 4]
, where
is an arbitrary constant.
In the long run, , The number of sheep will decrease and approach 0.
(iii) [Maximum marks: 2]
(iv) [Maximum marks: 4]
where
is an arbitrary constant.
(v) [Maximum marks: 2]
In the long run,
Note/ Comment: This question is quite difficult to interpret, but once you understand, it should be manageable.
(i) [Maximum marks: 3]
Let and
, then
(ii) [Maximum marks: 3]
Differentiate with respect to ,
Let
(iii) [Maximum marks: 1]
There are other external factors such as the strength of the kick, or wind, etc.
Note: I personally cannot think of a mathematical reason. Please share with me if you do. So I guess anything logical is fine.
(iv) [Maximum marks: 3]
.
When
(v) [Maximum marks: 2]

I wanna read the comment
you will have better luck at reddit
For the DE question, if i got the equation wrong but the steps in ii correct and deduced that overtime population approaches 0, will i get any mark.
you should. But the model will probably be wrong since math in general does not carry ecf.
does a wrong DE but correct way of solving DE get marks, thanks!
Given the marks distribution for the question, quite tough.. :/ Since the method to solve the DE was variable separable.
regarding question 2, if i did all steps correctly just during differentiation i carelessly left out the dy dx on the product side of the equation, will there be ecf? or will the 6 marks just fly away like that 🙁 thank you for your reply and the answers!
it won’t just fly away. if your method to find tangents, etc are correct. there will be marks.